Engineering Quantitative Aptitude English Reasoning General Knowledge Coding Others

Quiz: Permutations and Combinations

To View Tricks: Login Required

Number of Questions: 40

Question: 11 -

There are 30 people in a group. If all shake hands with one another , how many handshakes are possible?

Options:

  1. 30!

  2. 870

  3. 29! + 1

  4. 435

    5. Answer:

    435
    Solution:

    permutation and combination

Question: 12 -

In how many ways can we select 6 people out of 10, of which a particular person is not included?

Options:

  1. 9C4

  2. 9C5

  3. 10C3

  4. 9C6

    5. Answer:

    9C6
    Solution:

    One particular person is not included we have to select 6 persons out of 9 which can be done in 9C6 ways.

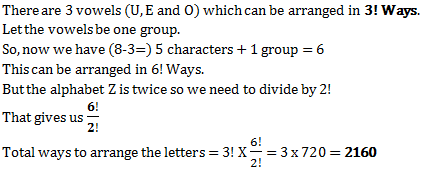
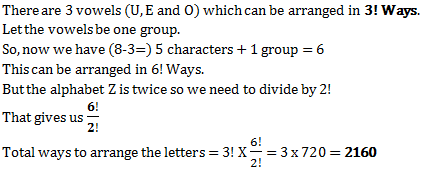
Question: 13 -

There are 20 points in a plane, how many triangles can be formed by these points if 5 are colinear?

Options:

  1. 1140

  2. 1130

  3. 1129

  4. 550

    5. Answer:

    1130
    Solution:

    Number of points in plane n = 20.
    Number of colinear points m = 5.
    Number of triangles from by joining n points of which m are colinear = nC3 – mC3
    Therefore the number of triangles = 20C3 – 5C3 = 1140-10 = 1130.

Question: 14 -

Find the number of rectangles and squares in an 8 by 8 chess board respectively.

Options:

  1. 204, 1092

  2. 296, 204

  3. 1092, 204

  4. 204, 1296

    5. Answer:

    1092, 204
    Solution:

    Chess board consists of 9 horizontal 9 vertical lines. A rectangle can be formed by any two horizontal and two vertical lines. Number of rectangles = 9C2 × 9C2 = 1296. For squares there is one 8 by 8 square four 7 by 7 squares, nine 6 by 6 squares and like this
    Number of squares on chess board = 12+22…..82 = 204
    Only rectangles = 1296-204 = 1092.

Question: 15 -

In how many ways can we arrange the word ‘FUZZTONE’ so that all the vowels come together?

Options:

  1. 6

  2. 4320

  3. 1440

  4. 2160

    5. Answer:

    2160
    Solution:

    permutation and combination