Question: 36 -
How many words, with or without meaning, can be formed using all letters of the word EQUATION using each letter exactly once?
-
38,400
-
39,320
-
38,320
-
40,320
Answer:
40,320
Solution:
The Word EQUATION has exactly 8 letters which are all different.
Therefore the number of words that can be formed = Number of permutations of 8 letters taken all at a time.
= P (8, 8) = 8!
= 8 × 7 x 6 × 5 x 4 x 3 x 2 × 1
= 40,320
The Word EQUATION has exactly 8 letters which are all different.
Therefore the number of words that can be formed = Number of permutations of 8 letters taken all at a time.
= P (8, 8) = 8!
= 8 × 7 x 6 × 5 x 4 x 3 x 2 × 1
= 40,320
Question: 37 -
Seven different objects must be divided among three people. In how many ways can this be done if one or two of them must get no objects?
-
84
-
381
-
36
-
180
Answer:
381
Solution:
Let the three people be A, B, and C.
If 1 person gets no objects, the 7 objects must be distributed such that each of the other two get 1 object at least.
This can be done as 6 & 1, 5 & 2, 4 & 3 and their rearrangements.
The answer would be ( 7C6 + 7C5 + 7C4 ) x 3! = 378
Also, two people getting no objects can be done in 3 ways.
Thus, the answer is 378 + 3 = 381
Let the three people be A, B, and C.
Question: 38 -
Ten participants are participating in a competition. In how many ways can the first three prizes be won?
-
920
-
720
-
680
-
820
Answer:
720
Solution:
Out of 10 participants, the first three prizes can be won in,
10P3 = 10! / (10 - 3)!
= 10 x 9 x 8
= 720 ways
Out of 10 participants, the first three prizes can be won in,
10P3 = 10! / (10 - 3)!
= 10 x 9 x 8
= 720 ways
Question: 39 -
A box contains 2 red balls, 3 black balls, and 4 white balls. Find the number of ways by which 3 balls can be drawn from the box in which at least 1 black ball should be present.
-
48
-
32
-
96
-
64
Answer:
64
Solution:
The possible combination could be (1 black ball and 2 non-black balls), (2 black balls and 1 non- black ball), and (only 3 black balls).
Therefore the required number of combinations = (3C1 * 6C2) + (3C2 * 6C1) + (3C3)
r,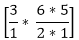 +
+ 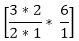 +
+ 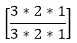 = 45+18+1 = 64
= 45+18+1 = 64
The possible combination could be (1 black ball and 2 non-black balls), (2 black balls and 1 non- black ball), and (only 3 black balls).
Therefore the required number of combinations = (3C1 * 6C2) + (3C2 * 6C1) + (3C3)
r,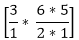 +
+ 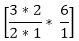 +
+ 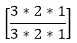 = 45+18+1 = 64
= 45+18+1 = 64
Question: 40 -
In how many ways can a cricketer can score 200 runs with fours and sixes only?
-
17
-
16
-
19
-
13
Answer:
17
Solution:
200 runs can be scored by scoring only fours or through a combination of fours and sixes.
Possibilities are 50 x 4, 47 x 4 + 2 x 6, 44 x 4 + 4 x 6 ... A total of 17 ways
200 runs can be scored by scoring only fours or through a combination of fours and sixes.
Possibilities are 50 x 4, 47 x 4 + 2 x 6, 44 x 4 + 4 x 6 ... A total of 17 ways